nLab third stable homotopy group of spheres

Idea

The third stable homotopy group of spheres (the third stable stem) is the cyclic group of order 24:

(1)$$\array{ \pi_3^s &\simeq& \mathbb{Z}/24 \\ [h_{\mathbb{H}}] &\leftrightarrow& [1] }$$

where the generator $[1] \in \mathbb{Z}/24$ is represented by the quaternionic Hopf fibration $S^7 \overset{h_{\mathbb{H}}}{\longrightarrow} S^4$.

from SS21

Properties

As the third framed bordism group

Under the Pontrjagin-Thom isomorphism, identifying the stable homotopy groups of spheres with the bordism ring $\Omega^{fr}_\bullet$ of stably framed manifolds (see at MFr), the generator (1) is represented by the 3-sphere (with its left-invariant framing induced from the identification with the Lie group SU(2) $\simeq$ Sp(1) )

$$\array{ \pi_3^s & \simeq & \Omega_3^{fr} \\ [h_{\mathbb{H}}] & \leftrightarrow & [S^3_{fr=1}] \,. }$$

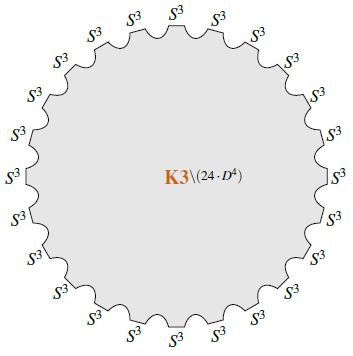
Moreover, the relation $24 \cdot [S^3_{Lie}] \,\simeq\, 0$ is represented by the bordism which is the complement of 24 open balls inside the K3-manifold (e.g. Wang-Xu 10, Sec. 2.6, Bauer 10, SP 17).

Via the fourth $(SU,fr)$-bordism group

Equivalently, the elements of $\pi_3^s \,\simeq\, \Omega^{fr}_3$ are detected by half the Todd classes of cobounding manifolds with special unitary group-tangential structure on their stable tangent bundle (elements of the MSUFr-bordism ring):

We have the following short exact sequence of the MSU-, MSUFr- and MFr-bordism rings (Conner-Floyd 66, p. 104)

(2)$$\array{ 0 \to & \Omega^{SU}_{8\bullet+4} & \overset{i}{\longrightarrow} & \Omega^{SU,fr}_{8\bullet+4} & \overset{\partial}{ \longrightarrow } & \Omega^{fr}_{8\bullet + 3} & \simeq & \pi^s_{8\bullet+3} \\ & \big\downarrow{}^{\tfrac{1}{2}\mathrlap{Td}} && \big\downarrow{}^{\tfrac{1}{2}\mathrlap{Td}} && \big\downarrow{}^{} && \big\downarrow{}^{e_{\mathbb{R}}} \\ 0 \to & \mathbb{Z} &\overset{\;\;\;\;\;}{\hookrightarrow}& \mathbb{Q} &\overset{\;\;\;\;}{\longrightarrow}& \mathbb{Q}/\mathbb{Z} &=& \mathbb{Q}/\mathbb{Z} }$$

which produces from half the Todd class of cobounding $(SU,fr)$-manifolds the KO-theoretic Adams e-invariant $e_{\mathbb{R}}$ (Adams 66, p. 39) of the boundary manifold in $\Omega^{fr}_{8k + 3} \simeq \pi^s_{8k+3}$. For $k = 0$ this detects the third stable homotopy group of spheres, by the following:

Proposition

(Adams 66, Example 7.17 and p. 46)

In degree 3, the KO-theoretic e-invariant $e_{\mathbb{R}}$ takes the value $\left[\tfrac{1}{24}\right] \in \mathbb{Q}/\mathbb{Z}$ on the quaternionic Hopf fibration $S^7 \overset{h_{\mathbb{H}}}{\longrightarrow} S^4$ and hence reflects the full third stable homotopy group of spheres:

$$\array{ \pi^s_3 & \underoverset{ \simeq }{ e_{\mathbb{R}} }{ \;\;\longrightarrow\;\; } & \mathbb{Z}/24 & \subset & \mathbb{Q}/\mathbb{Z} \\ [h_{\mathbb{H}}] &&\mapsto&& \left[\tfrac{1}{24}\right] }$$

while $e_{\mathbb{C}}$ sees only “half” of it (by Adams 66, Prop. 7.14).

Related concepts

• quaternionic Hopf fibration

• 24 branes transverse to K3

• first stable homotopy group of spheres

• second stable homotopy group of spheres

References

The original computation:

• Vladimir Abramovich Rokhlin, On a mapping of the $(n+3)$-dimensional sphere into the $n$-dimensional sphere, (Russian) Doklady Akad. Nauk SSSR (N.S.) 80, (1951). 541–544

with a mistake (in the unstable range) corrected in

• Vladimir Abramovich Rokhlin, New results in the theory of four-dimensional manifolds, (Russian) Doklady Akad. Nauk SSSR (N.S.) 84, (1952). 221–224.

French translations are in:

• Lucien Guillou, Alexis Marin (eds.), A la Recherche de la Topologie Perdue: I. Du côté de chez Rohlin. II. Le côté de Casson, Progress in Mathematics 62, Birkhäuser Boston 1985 (ISBN:0817633294, 9780817633295)

Review:

• Guozhen Wang, Zhouli Xu, Section 2.6 of: A survey of computations of homotopy groups of Spheres and Cobordisms, 2010 (pdf)

See also:

• Mehmet Kirdar, On the First, the Second and the Third Stems of the Stable Homotopy Groups of Spheres [arXiv:2107.06103]

More on the computation via the framed cobordism ring and the K3-manifold giving the cobordism that witnesses the order of 24:

• Tilman Bauer, answer to: third stable homotopy group of spheres via geometry?, 2010 (MO:a/44885)

• Chris Schommer-Pries, answer to: Nilpotence of the stable Hopf map via framed cobordism, 2017 (MO:a/218053)

Via immersions of 3-spheres into Euclidean 4-space

• A. Szűcs, Two Theorems of Rokhlin, Journal of Mathematical Sciences 113, 888–892 (2003) (doi:10.1023/A:1021208007146)

• Tobias Ekholm, Masamichi Takase, Singular Seifert surfaces and Smale invariants for a family of 3-sphere immersions, Bulletin of the London Mathematical Society 43 (2011) 251–266 (arXiv:0903.0238)

Discussion of geometric string bordism in degreee 3 as a means to speak (via the Pontryagin-Thom theorem) about the third stable homotopy group of spheres:

• Domenico Fiorenza, Eugenio Landi, Integrals detecting degree 3 string cobordism classes [arXiv:2209.12933]

• Domenico Fiorenza, String bordism invariants in dimension 3 from $U(1)$-valued TQFTs, talk at QFT and Cobordism, CQTS (Mar 2023) [web]